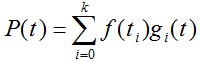拉格朗日(Lagrange)曲线插值
作者:追风剑情 发布于:2017-4-27 23:33 分类:Algorithms
已知若干个离散点的位置值和导数值,求经过这些点的插值多项式,是数学上熟知的Hermite插值问题。这一问题的明确提法是:已知函数f(t)在k+1个点{ti}处的函数值和导数值
这样的多项式P(t)就是对于函数f(t)的Hermite插值多项式。数学上已经证明,这样的多项式是存在且唯一的。
一、Lagrange插值公式
当
式中,gi(t)是混合函数。
使用此混合函数,使
任何类型的曲线都可以通过其控制点的线性组合来表示。
例如,当k=2,m0=m1=m2=1时,已知函数f(t)在三个点t0、t1和t2的函数值f(t0)、f(t1)和f(t2),则二次多项式P(t)为
式中,混合函数如下:
再如,设表示一条曲线的某个函数f(t)在四点
一般地,对于k+1个点
1)
2)
则
代码实现(C#版)
- using System;
- using System.Collections.Generic;
- using System.ComponentModel;
- using System.Data;
- using System.Drawing;
- using System.Linq;
- using System.Text;
- using System.Threading.Tasks;
- using System.Windows.Forms;
- namespace TestLine
- {
- public partial class Form1 : Form
- {
- public Form1()
- {
- InitializeComponent();
- }
- private void Form1_Load(object sender, EventArgs e)
- {
- }
- protected override void OnPaint(PaintEventArgs e)
- {
- LagrangeCurve curve = new LagrangeCurve();
- curve.input.Add(new Vector2(0, 0));
- curve.input.Add(new Vector2(10, 10));
- curve.input.Add(new Vector2(20, 20));
- curve.input.Add(new Vector2(30, 30));
- curve.input.Add(new Vector2(40, 40));
- curve.input.Add(new Vector2(50, 40));
- curve.input.Add(new Vector2(60, 30));
- curve.input.Add(new Vector2(70, 20));
- curve.input.Add(new Vector2(80, 10));
- curve.input.Add(new Vector2(90, 0));
- for (float x = 0; x <= 90; x++)
- {
- float y = curve.Lerp(x);
- PaintPoint(e, x, y);
- }
- }
- public void PaintPoint(PaintEventArgs e, float x, float y)
- {
- Graphics g = e.Graphics;
- Pen myPen = new Pen(Color.Red, 2);
- g.DrawEllipse(myPen, x, y, 2, 2);
- }
- }
- public class Vector2
- {
- public float x;
- public float y;
- public Vector2(float x, float y)
- {
- this.x = x;
- this.y = y;
- }
- }
- /// <summary>
- /// 拉格朗日曲线插值类
- /// </summary>
- public class LagrangeCurve
- {
- //输入点(即,曲线要经过的点)
- public List<Vector2> keyPoint = new List<Vector2>();
- public float Lerp(float x)
- {
- float y = 0;
- //构建input.Count个混合函数
- for (int i = 0; i < keyPoint.Count; i++)
- {
- float yi = keyPoint[i].y;
- float xi = keyPoint[i].x;
- float yj = 1;
- //每个混合函数需要遍历所有输入点(根据混合函数公式)
- for (int j = 0; j < keyPoint.Count; j++)
- {
- if (i != j)
- {
- float xj = keyPoint[j].x;
- yj *= (x - xj) / (xi - xj);
- }
- }
- y += yi * yj;
- }
- return y;
- }
- }
- }
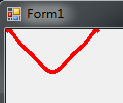
运行测试
标签: Algorithms
日历
最新文章
随机文章
热门文章
分类
存档
- 2025年3月(4)
- 2025年2月(3)
- 2025年1月(1)
- 2024年12月(5)
- 2024年11月(5)
- 2024年10月(5)
- 2024年9月(3)
- 2024年8月(3)
- 2024年7月(11)
- 2024年6月(3)
- 2024年5月(9)
- 2024年4月(10)
- 2024年3月(11)
- 2024年2月(24)
- 2024年1月(12)
- 2023年12月(3)
- 2023年11月(9)
- 2023年10月(7)
- 2023年9月(2)
- 2023年8月(7)
- 2023年7月(9)
- 2023年6月(6)
- 2023年5月(7)
- 2023年4月(11)
- 2023年3月(6)
- 2023年2月(11)
- 2023年1月(8)
- 2022年12月(2)
- 2022年11月(4)
- 2022年10月(10)
- 2022年9月(2)
- 2022年8月(13)
- 2022年7月(7)
- 2022年6月(11)
- 2022年5月(18)
- 2022年4月(29)
- 2022年3月(5)
- 2022年2月(6)
- 2022年1月(8)
- 2021年12月(5)
- 2021年11月(3)
- 2021年10月(4)
- 2021年9月(9)
- 2021年8月(14)
- 2021年7月(8)
- 2021年6月(5)
- 2021年5月(2)
- 2021年4月(3)
- 2021年3月(7)
- 2021年2月(2)
- 2021年1月(8)
- 2020年12月(7)
- 2020年11月(2)
- 2020年10月(6)
- 2020年9月(9)
- 2020年8月(10)
- 2020年7月(9)
- 2020年6月(18)
- 2020年5月(4)
- 2020年4月(25)
- 2020年3月(38)
- 2020年1月(21)
- 2019年12月(13)
- 2019年11月(29)
- 2019年10月(44)
- 2019年9月(17)
- 2019年8月(18)
- 2019年7月(25)
- 2019年6月(25)
- 2019年5月(17)
- 2019年4月(10)
- 2019年3月(36)
- 2019年2月(35)
- 2019年1月(28)
- 2018年12月(30)
- 2018年11月(22)
- 2018年10月(4)
- 2018年9月(7)
- 2018年8月(13)
- 2018年7月(13)
- 2018年6月(6)
- 2018年5月(5)
- 2018年4月(13)
- 2018年3月(5)
- 2018年2月(3)
- 2018年1月(8)
- 2017年12月(35)
- 2017年11月(17)
- 2017年10月(16)
- 2017年9月(17)
- 2017年8月(20)
- 2017年7月(34)
- 2017年6月(17)
- 2017年5月(15)
- 2017年4月(32)
- 2017年3月(8)
- 2017年2月(2)
- 2017年1月(5)
- 2016年12月(14)
- 2016年11月(26)
- 2016年10月(12)
- 2016年9月(25)
- 2016年8月(32)
- 2016年7月(14)
- 2016年6月(21)
- 2016年5月(17)
- 2016年4月(13)
- 2016年3月(8)
- 2016年2月(8)
- 2016年1月(18)
- 2015年12月(13)
- 2015年11月(15)
- 2015年10月(12)
- 2015年9月(18)
- 2015年8月(21)
- 2015年7月(35)
- 2015年6月(13)
- 2015年5月(9)
- 2015年4月(4)
- 2015年3月(5)
- 2015年2月(4)
- 2015年1月(13)
- 2014年12月(7)
- 2014年11月(5)
- 2014年10月(4)
- 2014年9月(8)
- 2014年8月(16)
- 2014年7月(26)
- 2014年6月(22)
- 2014年5月(28)
- 2014年4月(15)
友情链接
- Unity官网
- Unity圣典
- Unity在线手册
- Unity中文手册(圣典)
- Unity官方中文论坛
- Unity游戏蛮牛用户文档
- Unity下载存档
- Unity引擎源码下载
- Unity服务
- Unity Ads
- wiki.unity3d
- Visual Studio Code官网
- SenseAR开发文档
- MSDN
- C# 参考
- C# 编程指南
- .NET Framework类库
- .NET 文档
- .NET 开发
- WPF官方文档
- uLua
- xLua
- SharpZipLib
- Protobuf-net
- Protobuf.js
- OpenSSL
- OPEN CASCADE
- JSON
- MessagePack
- C在线工具
- 游戏蛮牛
- GreenVPN
- 聚合数据
- 热云
- 融云
- 腾讯云
- 腾讯开放平台
- 腾讯游戏服务
- 腾讯游戏开发者平台
- 腾讯课堂
- 微信开放平台
- 腾讯实时音视频
- 腾讯即时通信IM
- 微信公众平台技术文档
- 白鹭引擎官网
- 白鹭引擎开放平台
- 白鹭引擎开发文档
- FairyGUI编辑器
- PureMVC-TypeScript
- 讯飞开放平台
- 亲加通讯云
- Cygwin
- Mono开发者联盟
- Scut游戏服务器引擎
- KBEngine游戏服务器引擎
- Photon游戏服务器引擎
- 码云
- SharpSvn
- 腾讯bugly
- 4399原创平台
- 开源中国
- Firebase
- Firebase-Admob-Unity
- google-services-unity
- Firebase SDK for Unity
- Google-Firebase-SDK
- AppsFlyer SDK
- android-repository
- CQASO
- Facebook开发者平台
- gradle下载
- GradleBuildTool下载
- Android Developers
- Google中国开发者
- AndroidDevTools
- Android社区
- Android开发工具
- Google Play Games Services
- Google商店
- Google APIs for Android
- 金钱豹VPN
- TouchSense SDK
- MakeHuman
- Online RSA Key Converter
- Windows UWP应用
- Visual Studio For Unity
- Open CASCADE Technology
- 慕课网
- 阿里云服务器ECS
- 在线免费文字转语音系统
- AI Studio
- 网云穿
- 百度网盘开放平台
- 迅捷画图
- 菜鸟工具
- [CSDN] 程序员研修院
- 华为人脸识别
- 百度AR导航导览SDK
- 海康威视官网
- 海康开放平台
- 海康SDK下载
- git download
- Open CASCADE
- CascadeStudio
交流QQ群
-
Flash游戏设计: 86184192
Unity游戏设计: 171855449
游戏设计订阅号